Difference between revisions of "SpringSemester"

From Laboratory Courses at the Automatic Control Lab
Jump to navigation Jump to search
Line 9: Line 9:
 
----
 
----
 
==== 2.2 Self Erecting Inverted Pendulumg - LQR ====
 
==== 2.2 Self Erecting Inverted Pendulumg - LQR ====
 +
 +
[[File:SEIP.jpg|right|frame|200px|Self Erecting Inverted Pendulum - LQR]]
 +
In this experiment, a pendulum is mounted on a cart. The pendulum shall be controlled to stay in its unstable equilibrium, i.e. the upright position. You will design an LQR controller to achieve this goal. Additionally, you will implement a destabilizing controller that will make the pendulum swing up from its stable downward position. Finally, the two controllers will be combined to yield a self-erecting pendulum.
 +
 +
====== Prerequisites ======
 +
* Lead/Lag Compensators
 +
 +
====== Homework ======
 +
Preparation time approx 2.5 hrs, see Manual.
 +
 +
====== Place ======
 +
[http://www.rauminfo.ethz.ch/Rauminfo/RauminfoPre.do?region=Z&areal=Z&gebaeude=ETL&geschoss=D&raumNr=12 ETL D12]
 +
 +
====== Downloads ======
 +
[[media:IfA_2-2_manual.pdf|Manual]]
 +
 +
[[media:IfA_2-2_manual.pdf|Matlab Template]]
 +
 +
  
 
----
 
----
 
==== 2.6 Helicopter II - Lead/Lag ====
 
==== 2.6 Helicopter II - Lead/Lag ====
 +
 +
[[File:HelicopterII.jpg|right|frame|200px|Helicopter II - Lead/Lag]]
 +
You will control the two coupled axes of a helicopter model. First the model of the plant is calculated and then linearized. Using Matlab and Simulink, you will design a compensation controller (Lead/Lag), which can then be tested on the real system.
 +
 +
====== Prerequisites ======
 +
* Lead/Lag Compensators
 +
 +
====== Homework ======
 +
Preparation time approx 2.5 hrs, see Manual.
 +
 +
====== Place ======
 +
[http://www.rauminfo.ethz.ch/Rauminfo/RauminfoPre.do?region=Z&areal=Z&gebaeude=ETL&geschoss=D&raumNr=12 ETL D12]
 +
 +
====== Downloads ======
 +
[[media:IfA_2-6_manual.pdf|Manual]]
 +
 +
[[media:IfA_2-6_manual.pdf|Matlab Template]]
 +
 +
  
 
----
 
----
 
==== 3.4 Quad Tank ====
 
==== 3.4 Quad Tank ====
 +
 +
[[File:QuadTank.jpg|right|frame|200px|Quad Tank]]
 +
The quad-tank system is a relatively simple MIMO (multi-input, multi-output) system. MIMO systems are inherently more difficult to control than systems with only one input / output.
 +
In this experiment, you will learn some fundamental techniques to control a MIMO system, like coupled- and decoupled designs or LQR / LQG state-space controllers.
 +
 +
====== Prerequisites ======
 +
* Basics in MIMO control
 +
* Minimum/Non-minimum phase plants
 +
* PI control
 +
* LQR control
 +
 +
====== Homework ======
 +
Preparation time approx 2.5 hrs, see Manual.
 +
 +
====== Place ======
 +
[http://www.rauminfo.ethz.ch/Rauminfo/RauminfoPre.do?region=Z&areal=Z&gebaeude=ETL&geschoss=D&raumNr=12 ETL D12]
 +
 +
====== Downloads ======
 +
[[media:IfA_3-4_manual.pdf|Manual]]
 +
 +
 +
  
 
----
 
----
 
==== 3.7A Flexible Shaft A - System Analysis & PI ====
 
==== 3.7A Flexible Shaft A - System Analysis & PI ====
 +
 +
[[File:FlexShaft.jpg|right|frame|200px|Flexible Shaft A - System Analysis & PI]]
 +
This experiment emphasizes the properties of so-called flexible structures. These are mechanical systems that allow to be modeled as a system of many masses interlinked by springs. Examples are robots, huge satellite dishes, power trains and so on. The experiment itself consists of a shaft with two inertia wheels that are connected by a spring. A drive motor accelerates the shaft whereas a load motor is used to simulate a load torque. The student starts with a system analysis of this setup and investigates typical resonance and anti-resonance behavior which also get interpreted in terms of pole/zero configurations. Afterwards, a PI controller get designed to control the speed of the shaft.
 +
 +
====== Prerequisites ======
 +
* PID Controller (RS1 § 4,12; week 4 and 8)
 +
 +
====== Homework ======
 +
Preparation time approx 2 hrs, see Manual.
 +
 +
====== Place ======
 +
[http://www.rauminfo.ethz.ch/Rauminfo/RauminfoPre.do?region=Z&areal=Z&gebaeude=ETL&geschoss=D&raumNr=12 ETL D12]
 +
 +
====== Downloads ======
 +
[[media:IfA_3-7A_manual.pdf|Manual]]
 +
 +
[[media:IfA_3-7A_manual.pdf|Matlab Template]]
 +
 +
  
 
----
 
----
 
==== 3.7B Flexible Shaft B - Loop Shaping & LQR ====
 
==== 3.7B Flexible Shaft B - Loop Shaping & LQR ====
 +
 +
[[File:FlexShaft.jpg|right|frame|200px|Flexible Shaft A - System Analysis & PI]]
 +
This experiment is the continuation of experiment 3.7A. Here, students design two more feedback controllers to control the speed of the flexible shaft. The first controller is obtained from a loop shaping procedure. The second controller consists of a state-feedback LQ controller with integral action and an appropriate observer. Please note that the experiment 3.7A has to be done prior to this one.
 +
 +
====== Prerequisites ======
 +
* Experiment 3.7A completed
 +
* LQR (RS1 § 17.9; week 13)
 +
 +
====== Homework ======
 +
Preparation time approx 2 hrs, see Manual.
 +
 +
====== Place ======
 +
[http://www.rauminfo.ethz.ch/Rauminfo/RauminfoPre.do?region=Z&areal=Z&gebaeude=ETL&geschoss=D&raumNr=12 ETL D12]
 +
 +
====== Downloads ======
 +
[[media:IfA_3-7B_manual.pdf|Manual]]
 +
 +
[[media:IfA_3-7B_manual.pdf|Matlab Template]]
 +
 +
  
 
----
 
----
  
 
</div>
 
</div>

Revision as of 09:32, 25 March 2014

Experiments in the Spring Semester

On this page you will find short descriptions of every experiment we offer. You can also download the manuals and necessary files for your homework preparation from here.

Registration: Please register for experiments on the D-ITET online registration website.


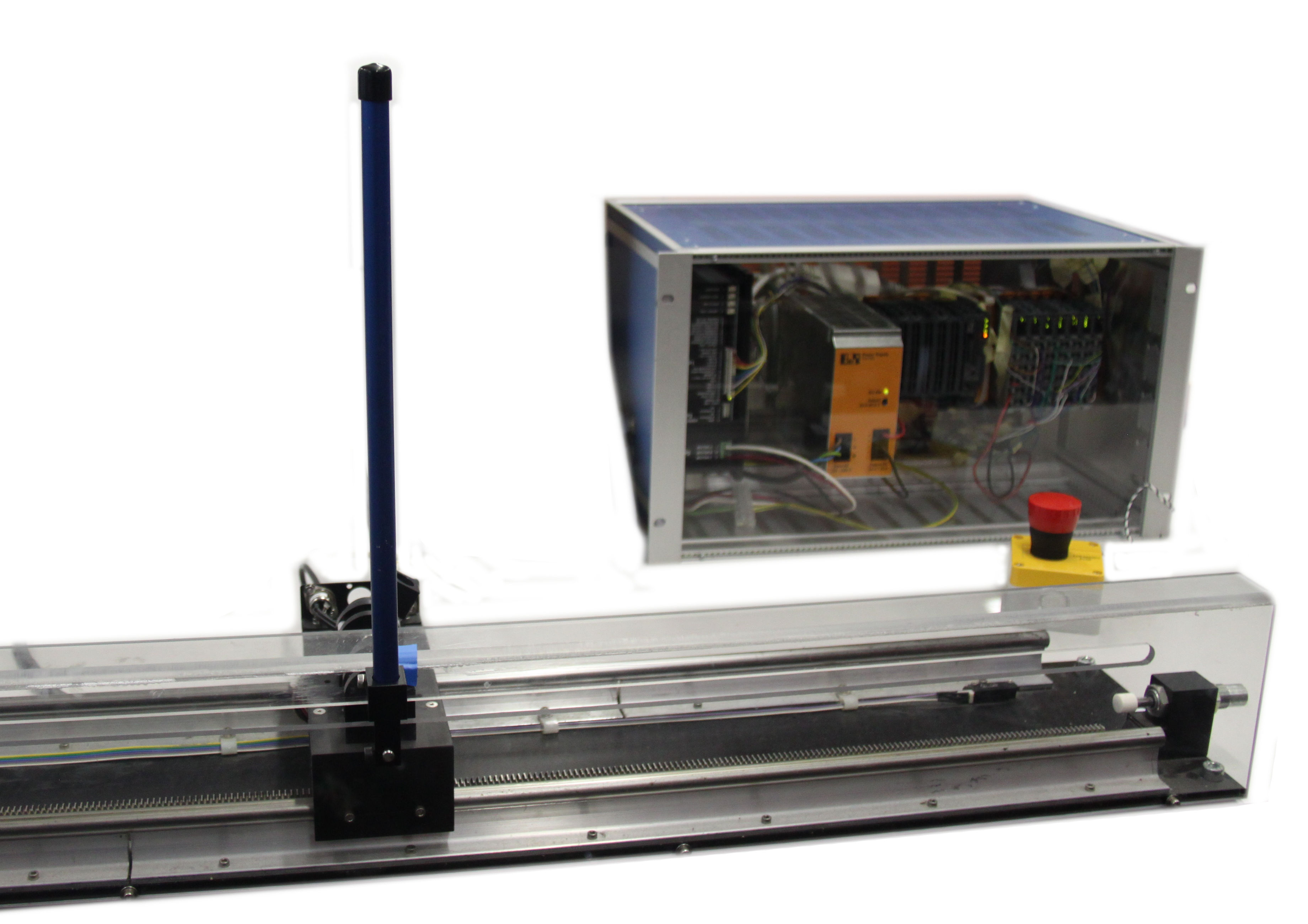
2.2 Self Erecting Inverted Pendulumg - LQR

Self Erecting Inverted Pendulum - LQR

In this experiment, a pendulum is mounted on a cart. The pendulum shall be controlled to stay in its unstable equilibrium, i.e. the upright position. You will design an LQR controller to achieve this goal. Additionally, you will implement a destabilizing controller that will make the pendulum swing up from its stable downward position. Finally, the two controllers will be combined to yield a self-erecting pendulum.

Prerequisites
  • Lead/Lag Compensators
Homework

Preparation time approx 2.5 hrs, see Manual.

Place

ETL D12

Downloads

Manual

Matlab Template


2.6 Helicopter II - Lead/Lag

Helicopter II - Lead/Lag

You will control the two coupled axes of a helicopter model. First the model of the plant is calculated and then linearized. Using Matlab and Simulink, you will design a compensation controller (Lead/Lag), which can then be tested on the real system.

Prerequisites
  • Lead/Lag Compensators
Homework

Preparation time approx 2.5 hrs, see Manual.

Place

ETL D12

Downloads

Manual

Matlab Template


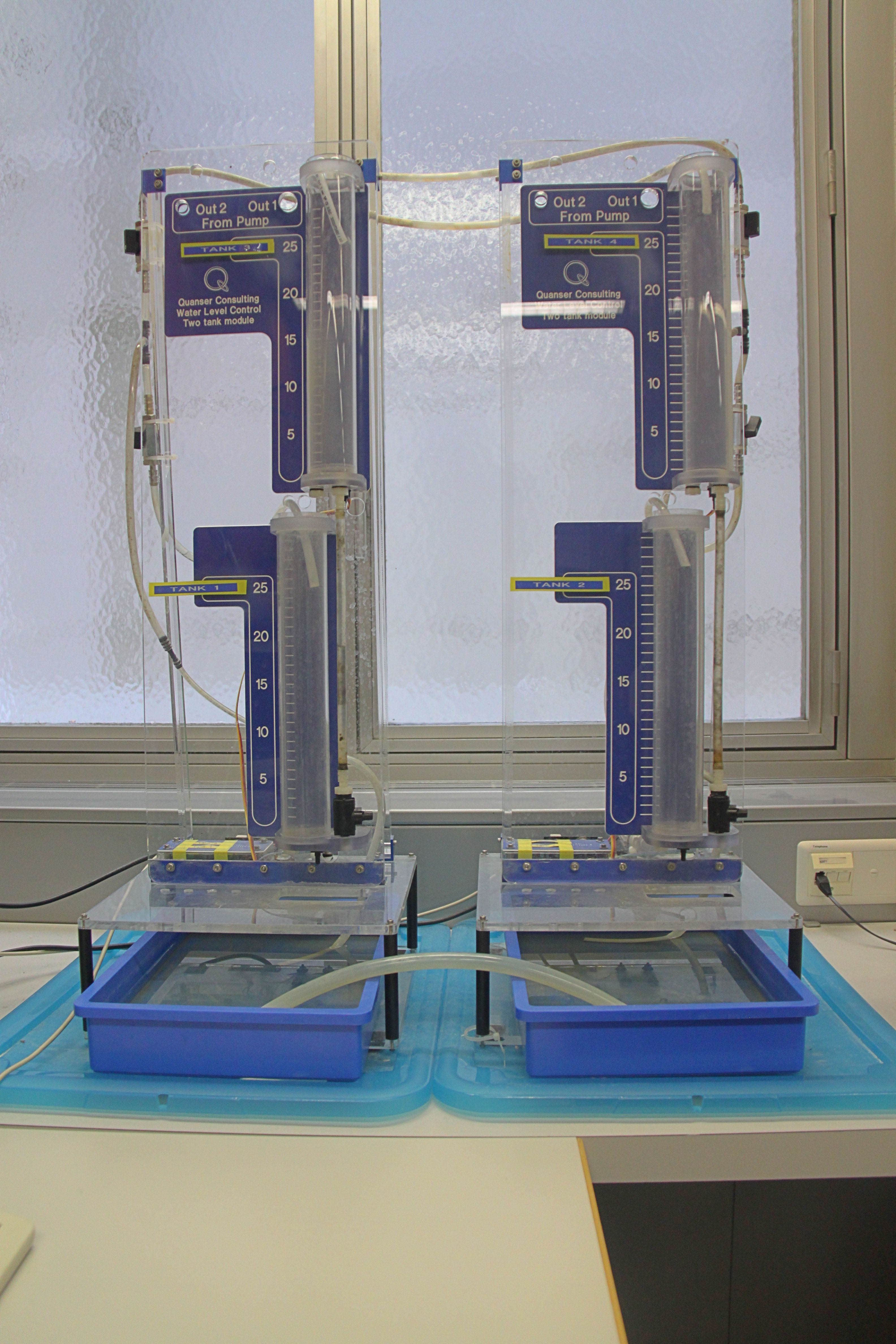
3.4 Quad Tank

Quad Tank

The quad-tank system is a relatively simple MIMO (multi-input, multi-output) system. MIMO systems are inherently more difficult to control than systems with only one input / output. In this experiment, you will learn some fundamental techniques to control a MIMO system, like coupled- and decoupled designs or LQR / LQG state-space controllers.

Prerequisites
  • Basics in MIMO control
  • Minimum/Non-minimum phase plants
  • PI control
  • LQR control
Homework

Preparation time approx 2.5 hrs, see Manual.

Place

ETL D12

Downloads

Manual



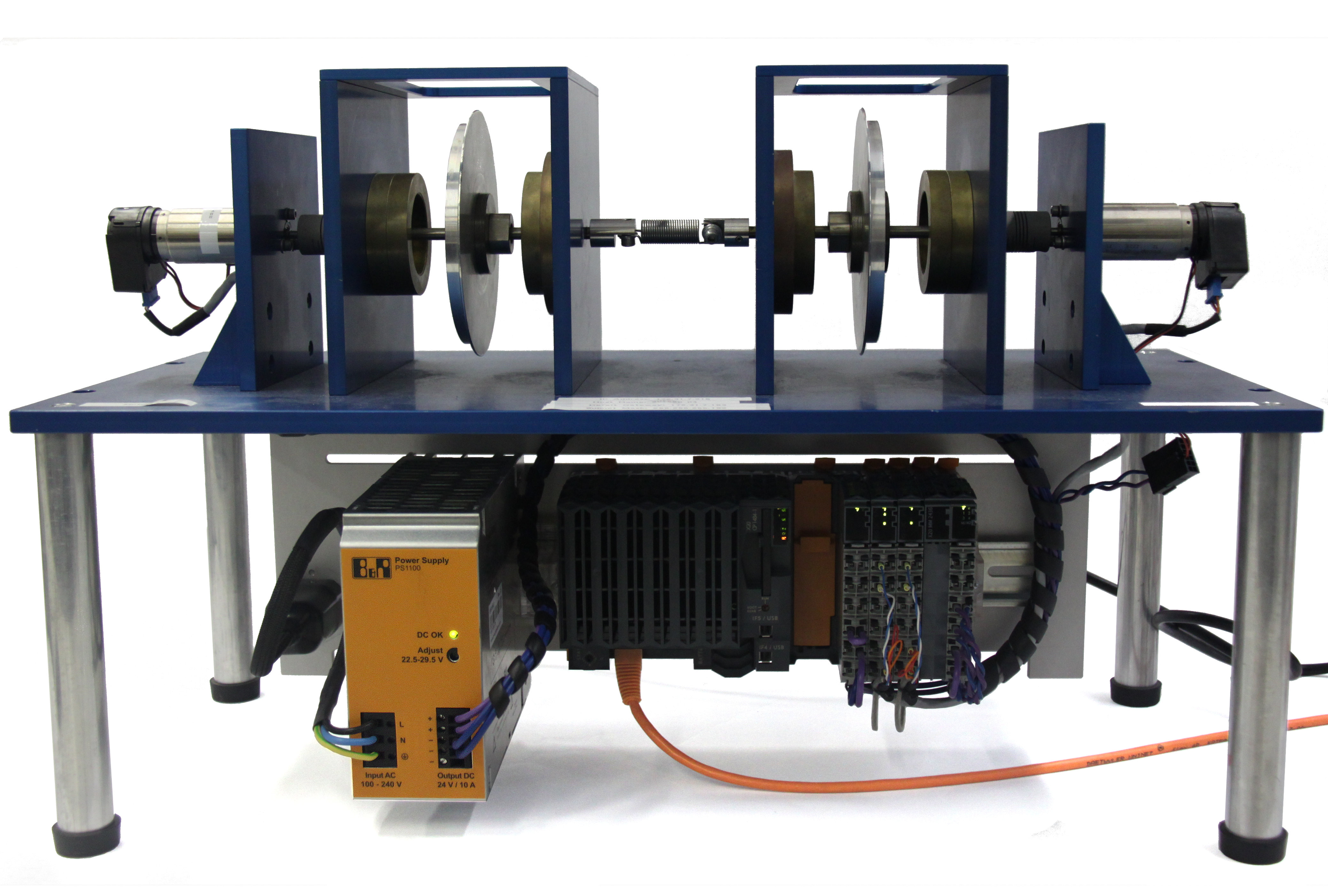
3.7A Flexible Shaft A - System Analysis & PI

Flexible Shaft A - System Analysis & PI

This experiment emphasizes the properties of so-called flexible structures. These are mechanical systems that allow to be modeled as a system of many masses interlinked by springs. Examples are robots, huge satellite dishes, power trains and so on. The experiment itself consists of a shaft with two inertia wheels that are connected by a spring. A drive motor accelerates the shaft whereas a load motor is used to simulate a load torque. The student starts with a system analysis of this setup and investigates typical resonance and anti-resonance behavior which also get interpreted in terms of pole/zero configurations. Afterwards, a PI controller get designed to control the speed of the shaft.

Prerequisites
  • PID Controller (RS1 § 4,12; week 4 and 8)
Homework

Preparation time approx 2 hrs, see Manual.

Place

ETL D12

Downloads

Manual

Matlab Template


3.7B Flexible Shaft B - Loop Shaping & LQR

Flexible Shaft A - System Analysis & PI

This experiment is the continuation of experiment 3.7A. Here, students design two more feedback controllers to control the speed of the flexible shaft. The first controller is obtained from a loop shaping procedure. The second controller consists of a state-feedback LQ controller with integral action and an appropriate observer. Please note that the experiment 3.7A has to be done prior to this one.

Prerequisites
  • Experiment 3.7A completed
  • LQR (RS1 § 17.9; week 13)
Homework

Preparation time approx 2 hrs, see Manual.

Place

ETL D12

Downloads

Manual

Matlab Template


Retrieved from "http://people.ee.ethz.ch/~ifa-fp/wikimedia/index.php?title=SpringSemester&oldid=48"