PCS0x0.PRO: Photonic Crystal
Band diagram of a perfect photonic crystal,
Photonic band gap, 2D MMP, Advanced eigenvalue search,
Periodic structure
J. Smajic, Computational Optics Group, IFH, ETH,
8092
Back to MaX-1, MaX-1 Examples Overview
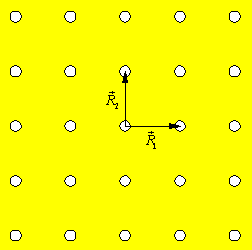
Fig. 1. Photonic crystal structure (original lattice) with two lattice vectors
In the description of the 2D photonic crystal with triangular lattice, all details about reciprocal lattice, the 1st Brillouin zone construction and periodic constants calculation are given. Following this background, the results of these calculations are presented here.
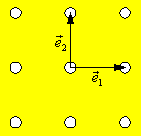
The primitive lattice vectors for the square lattice are shown in the following figures.
|
Fig. 2. The original lattice |
Fig. 3. The reciprocal lattice |

Fig. 4. The 1st Brollouin zone construction and detail of the irreducible part
Example:
Let us consider a dielectric 2D photonic crystal with: a = 10-6 (m), r = 0.18a, εr = 11.56 (inside of the dielectric rods), square lattice (Fig. 1.). The wave vector components and periodic constants are:
![]() , and
, and ![]() ,
,
The results of the periodic constant calculation for the three corners M, K, and Г of the irreducible 1st BZ are:
Г: ![]() ,
,
![]() ,
,
![]() ,
, ![]() ,
,
M: ![]() ,
, ![]() ,
,
![]() ,
, ![]() ,
,
X: ![]() ,
, ![]() ,
,
![]() ,
, ![]() .
.
The results of the MaX-1 bandgap computations are given in the Fig. 8. and Fig. 9.
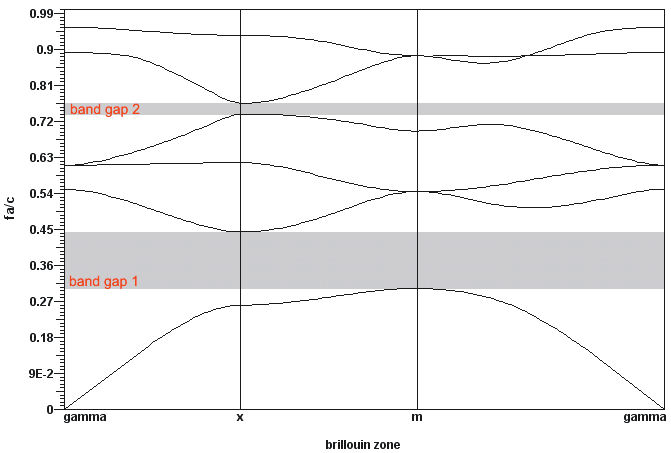
Fig. 7. Band structure of the 2D dielectric photonic crystal for the TE modes
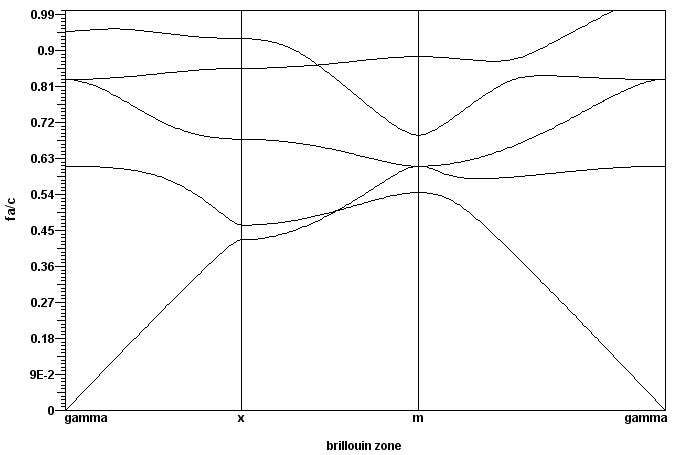
Fig. 8. Band structure of the 2D dielectric photonic crystal for the TM modes
Download the project for TE and TM modes! Back to my home page!